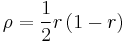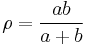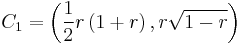Archimedes' twin circles
In geometry, Archimedes' circles, first created by Archimedes, are two circles that can be created inside of an arbelos, both having the same area as each other.
Contents |
Construction
From any three collinear points A, B, and C, one may form an arbelos, a shape bounded by three semicircles having pairs of these three points as their diameters. All three semicircles must be on the same side of line AC. Archimedes' twin circles are created by drawing a perpendicular line to line AC through the middle point B of the three given points, tangent to the two smaller semicircles. Each of the two circles C1 and C2 is tangent to that line and to the large semicircle; C1 is tangent to one of the smaller semicircles and C2 is tangent to the other smaller semicircle. Each of the two circles is uniquely determined by its three tangencies; constructing each of the twin circles from its tangencies is a special case of the Problem of Apollonius.
Radii of the circles
Because the two circles are congruent, they both share the same radius length. If r = AB/AC, then the radius of either circle is:
Also, according to Proposition 5 of Archimedes' Book of Lemmas, the common radius of any Archimedean circle is:
where a and b are the radii of two inner semicircles.
Centers of the circles
If r = AB/AC, then the centers to C1 and C2 are:
See also
References
- Weisstein, Eric W. ""Archimedes' Circles." From MathWorld--A Wolfram Web Resource". http://mathworld.wolfram.com/ArchimedesCircles.html. Retrieved 2008-04-10.